Grade 11 Measures of Dispersion Exercise 1 Solutions | Basic Mathematics Grade XI by Sukunda Pustak Bhawan

In this page, you can find the complete solutions of the first exercise of Measures of Dispersion chapter from Basic Mathematics Grade XI book published and distributed by Sukunda Pustak Bhawan.

In the above-mentioned book, sequence and series is the 14th chapter and has two exercises only. Out of which, this is the solution of the first exercise in which we have to deal with the mean, standard deviation, and coefficient of variation.

Related Solutions:

Grade 11 Basic Mathematics by Sukunda Pustak Vawan Notes and Solutions | Nepal

Grade 11 Sequence and Series Exercise 1 Solutions | Basic Mathematics Grade XI by Sukunda Pustak Bhawan

Disclaimer:

Answers mentioned here are not solved by teachers. These are the solutions written by a student of Grade 11. Answers are all correct. However, the language or process of solving the questions might be informal and in examinations, you might have to add little more language and show more calculations than what has been done here. So, we highly encourage you to view these solutions as guide rather than just copying everything mentioned here. Few questions have been typed while most of them have been updated as pictures.

Unit 14 Measures of Dispersion

Part 2 Methods of measuring dispersion: Standard Deviation

---

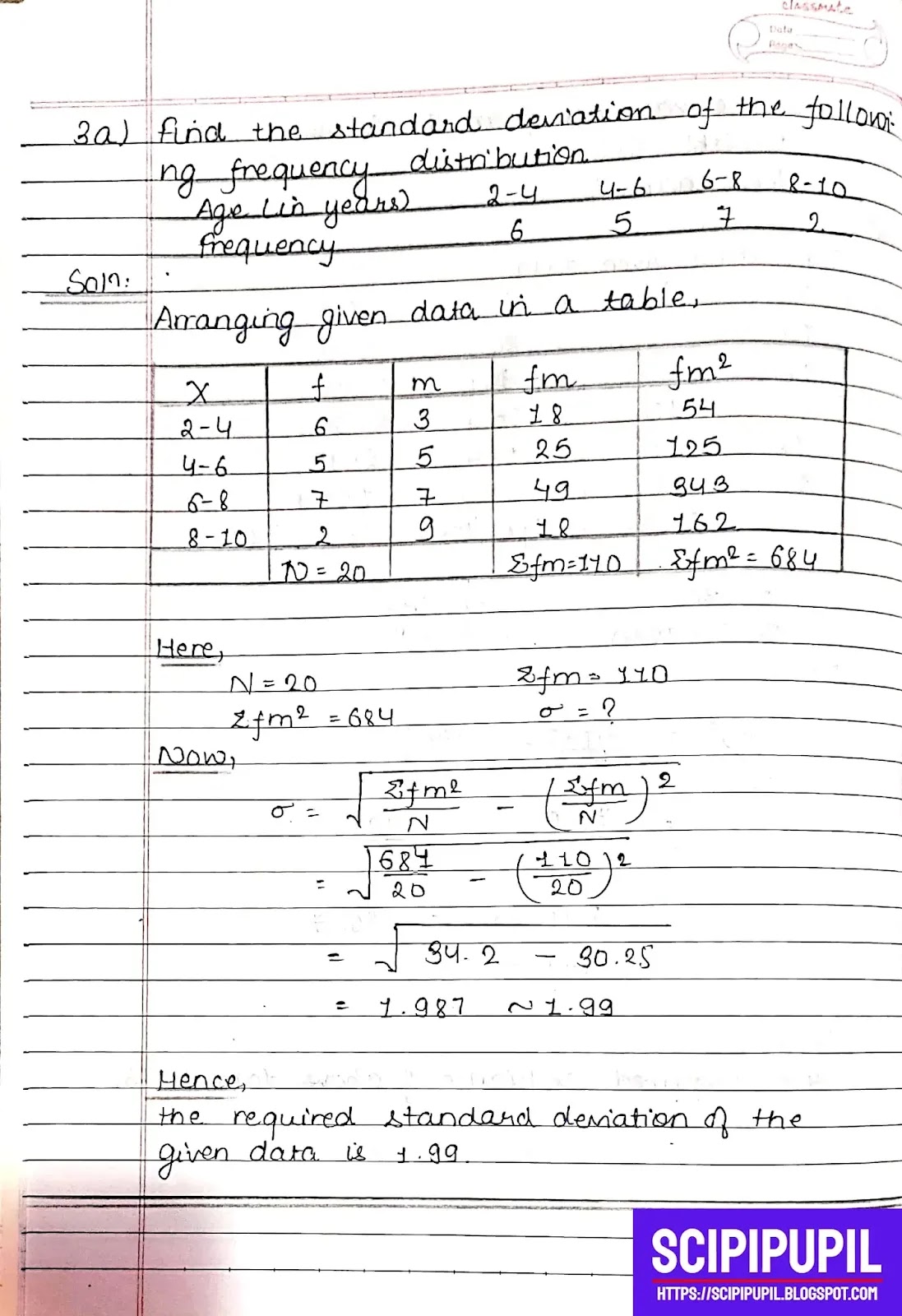
1 a) Find the standard deviation from the following set of observations.

20 25 30 36 32 43

Solution: Arranging the given data in a table and computing the values,

$x$	$x^2$
20	400
25	625
30	900
36	1296
32	1024
43	1849
$\sum x = 186$	$\sum x^2 = 6094$

Here,

$\sum x = 186$

$\sum x^2 = 6094$

$n = 6$

$\sigma = ?$

We know,

$\sigma = \sqrt{ \dfrac{ \sum x^2}{n}  - \left ( \dfrac{ \sum fx}{n} \right )^2 }$

$= \sqrt{ \dfrac{6094}{6} - \left ( \dfrac{186}{6} \right )^2}$

$= \sqrt{1015.66 - 31^2}$

$= 7.39$

Hence, the required standard deviation of the given data is 7.39.



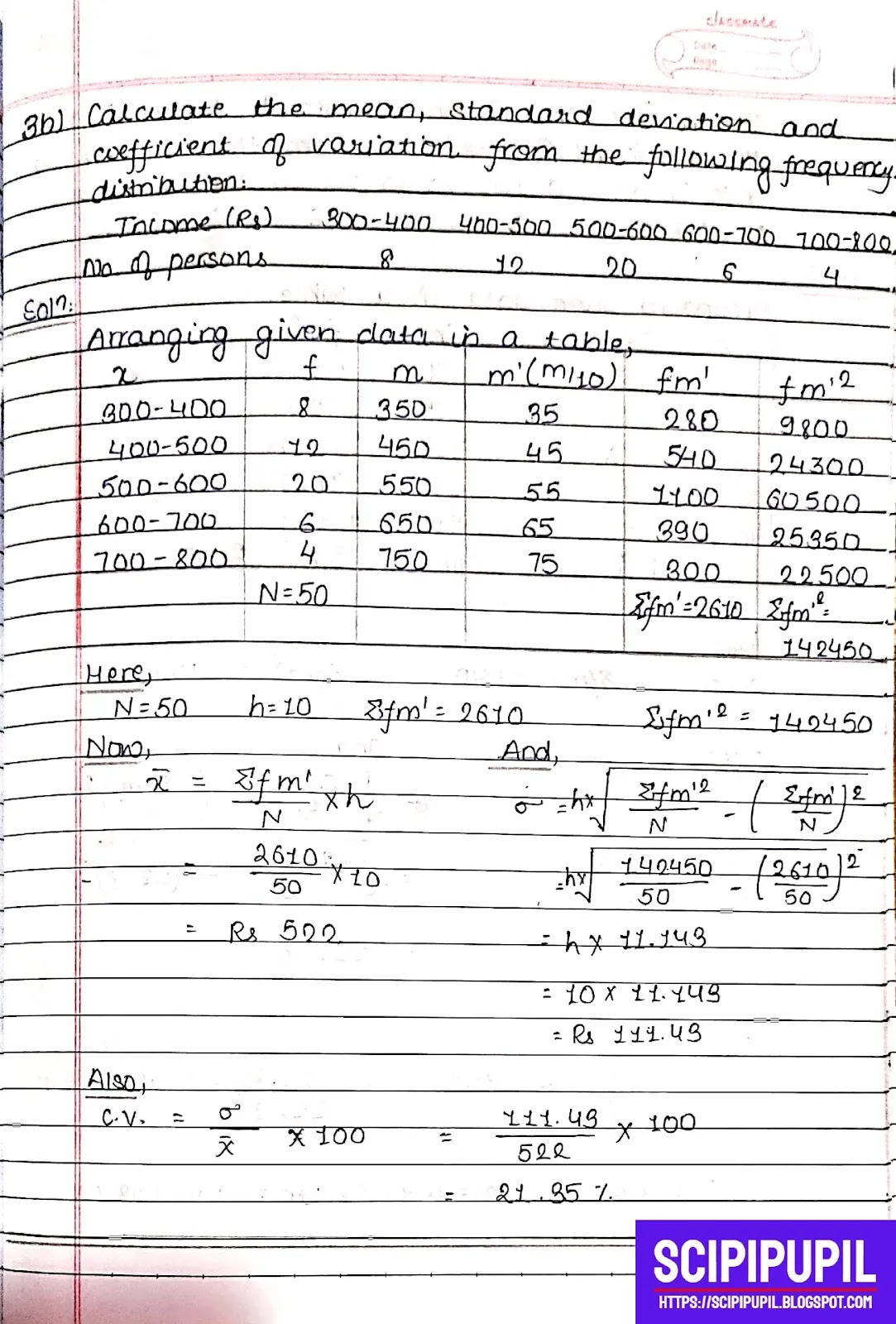
1 b) Daily expenditure of 6 families are given below:

Rs 240, Rs 180, Rs 320, Rs 160, Rs 260, Rs 400

Find the standard deviation.

Solution: Arranging the given data in a table and computing the values,

$x$	$x^2$
240	57600
180	32400
320	102400
160	25600
260	67600
400	160000
$\sum x = 1560$	$\sum x^2 = 445600$

Here,

$\sum x = 1560$

$\sum x^2 = 445600$

$n = 6$

$\sigma = ?$

We know,

$\sigma = \sqrt{ \dfrac{ \sum x^2}{n}  - \left ( \dfrac{ \sum fx}{n} \right )^2 }$

$= \sqrt{ \dfrac{445600}{6} - \left ( \dfrac{1560}{6} \right )^2}$

$= \sqrt{75266.67 - 260^2}$

$= 81.65$

Hence, the required standard deviation of the given data is 81.65.

---

---

---

---

---

---

---

---

---

---

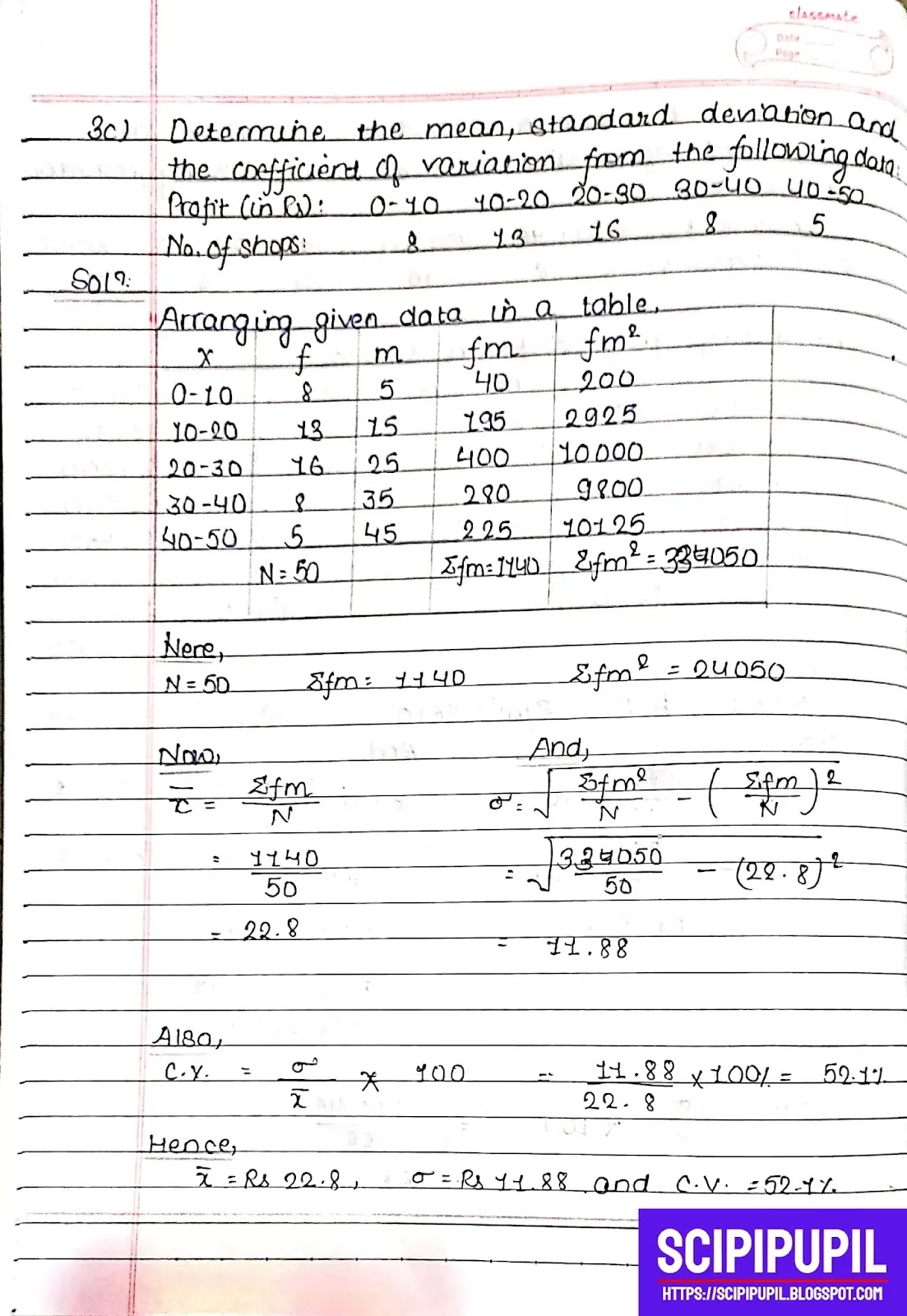
6 a) Following are the marks obtained by the students X and Y in 6 tests of 100 makrs each:

Test	1	2	3	4	5	6
X	56	72	48	69	64	81
Y	63	74	45	57	82	63

If the consistency of the performance is the criteria for awarding a prize, who should get the prize?

Solution:

The lower the coefficient of variation, the more the consistency is.

Here, $n = 6$ for both X and Y.

Computing mean and standard deviation of student X

$x$	$x^2$
56	3136
72	5184
48	2304
69	4761
64	4096
81	6561
$\sum x = 390$	$\sum x^2 = 26042$

$\sum x = 390$

$\sum x^2 = 26042$

$n = 6$

$\sigma = ?$

We know,

$\sigma_X = \sqrt{ \dfrac{ \sum x^2}{n}  - \left ( \dfrac{ \sum fx}{n} \right )^2 }$

$= \sqrt{ \dfrac{26042}{6} - \left ( \dfrac{390}{6} \right )^2}$

$= 10.74$

$\overline{x}_X = \dfrac{ \sum x}{n}$

$= \dfrac{ 390}{6}$

$= 65$

$\therefore C.V._X  = \dfrac{ \sigma _X}{ \overline{x} _X} * 100$

$= \dfrac{10.74}{65} * 100$

$= 16.52 \%$

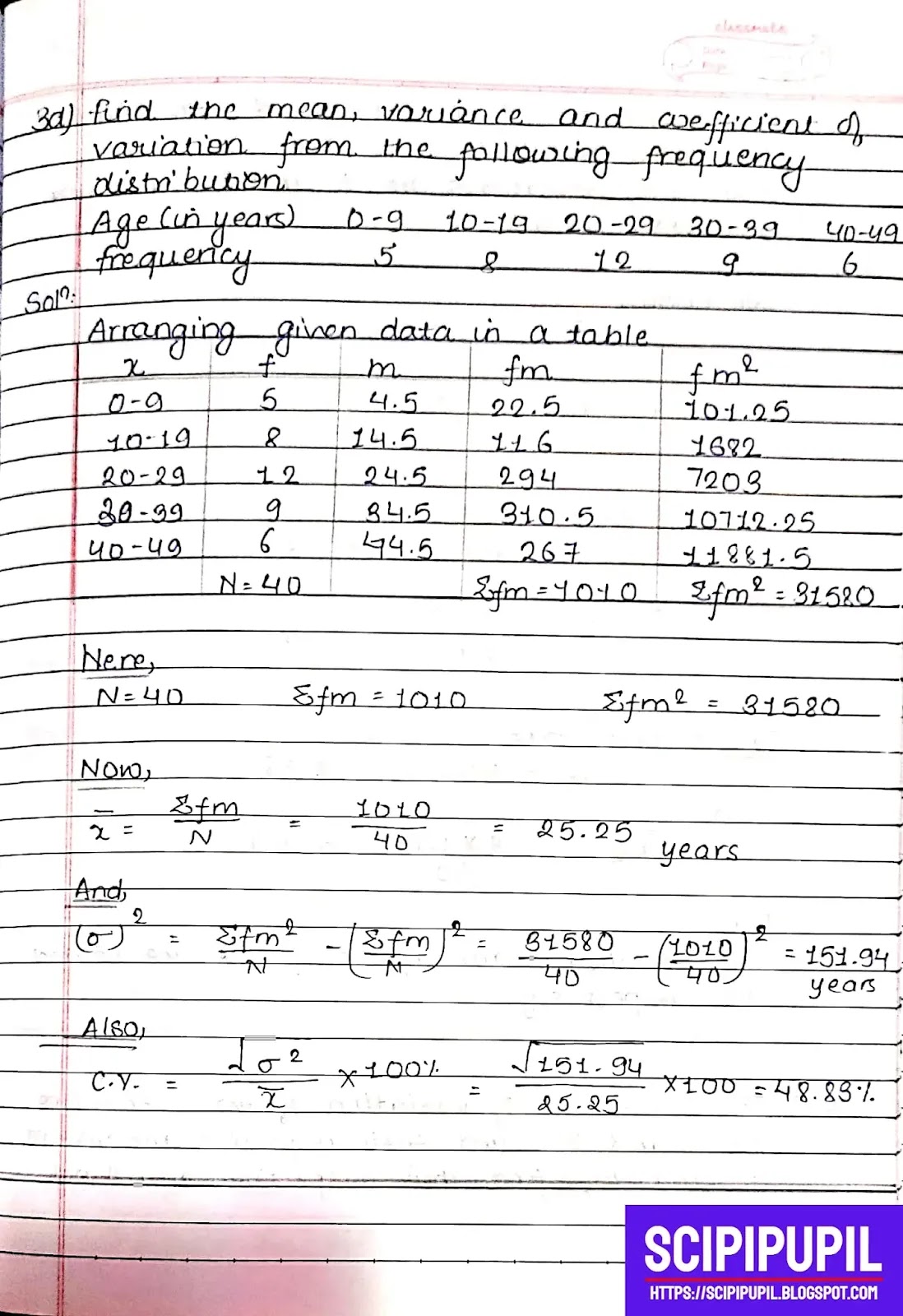
Computing mean and standard deviation of student Y

$x$	$x^2$
63	3969
74	5476
45	2025
57	3249
82	6724
63	3969
$\sum x = 384$	$\sum x^2 = 25412$

$\sum x = 384$

$\sum x^2 = 25412$

$n = 6$

$\sigma = ?$

We know,

$\sigma_Y = \sqrt{ \dfrac{ \sum x^2}{n}  - \left ( \dfrac{ \sum fx}{n} \right )^2 }$

$= \sqrt{ \dfrac{25412}{6} - \left ( \dfrac{384}{6} \right )^2}$

$= 11.80$

$\overline{x}_Y = \dfrac{ \sum x}{n}$

$= \dfrac{ 384}{6}$

$= 64$

$\therefore C.V._X  = \dfrac{ \sigma _Y}{ \overline{x}_Y} * 100$

$= \dfrac{11.80}{64} * 100$

$= 18.44 \%$

Since, Coefficient of Variation of student X is less than that of student Y, student X should get the prize for his better consistency.

---

7 b) The monthly wages paid to the workers of two firms A and B belonging to the same industry have been presented below.

	Firm A	Firm B
No. of workers	50	40
Average monthly wage	Rs. 63	Rs. 54
Variance of wages	81	36

Determine the combined mean and combined standard deviation of the combined group of 90 workers.

Solution:

For Firm A,

$n_1 = 50$

$\overline{x}_1 = Rs. 63$

$\sigma _1^2 = 81$

For Firm B,

$n_2 = 40$

$\overline{x}_1 = Rs. 54$

$\sigma _1^2 = 36$

Now,

Combined mean $\overline{x}_{12}$ $= \dfrac{n_1 \overline{x}_1  + n_2 \overline{x}_2}{n_1 + n_2}$

$= \dfrac{50 * 63 + 40 * 54}{50 + 40}$

$= \dfrac{5310}{90}$

$= 59$

And,

$d_1 = x_1 - x_{12}$

$\therefore d_1 = 63 - 59 = 4$

$d_2 = x_{12} - x_2$

$\therefore d_2 = 59 - 54 = 5$

$\sigma _{12} = \sqrt{ \dfrac{n_1 ( d_1^2 + \sigma _1^2) + n_2 (d_2^2 + \sigma _2^2) }{n_1 + n_2} }$

$= \sqrt{ \dfrac{50 (81 + 16) + 40 (36 + 25)}{50+ 40} }$

$= \sqrt{ \dfrac{50 * 97 + 40 *61}{90}}$

$= 9$

---

8 a) The arithmetic mean and the standard deviation of a series of 20 items as calculated by a student were 20 cm and 5 cm resepectively. But while calculating an item 13 was misread as 30. Find the correct mean and standard deviation.

Solution:

Here,

Total number of observations (n) = 20

Inaccurate mean ($\overline{x}_1$) = 20 cm

Inaccurate standard deviation ($\sigma_1$) = 5 cm

Given,

13 was misread as 30

Now,

Total sum of observations in in accurate mean ($\sum x_1$) = $\overline{x}_1 * n$

$= 20 * 20$

$= 400$

Since 13 was misread as 30,

Total sum of observations after correcting ($\sum x_2$) = $400 - 30 + 13$

$= 383$

Accurate mean $\overline{x}_2$ = $\dfrac{ \sum x_2}{n}$

$= \dfrac{383}{20}$

$= 19.15 \text{cm}$

And,

$(\sigma_1)^2 = \dfrac{ \sum x_1^2}{n} - \left ( \dfrac{ \sum x_1 }{n} \right )^2$

$or, 5^2 = \dfrac{ \sum x_1^2}{20}  - (20)^2$

$or, 425 * 20 = \sum x_1^2$

$\implies \sum x_1^2 = 8500$

Since 13 was misread was 30

Accurate sum of the square of the observations ($\sum x_2^2$) = $8500 - 30^2 + 13^2$

$= 7769$

Again,

Accurate standard deviation of the given data is

$\sigma _ 2 = \sqrt{ \dfrac{ \sum x_2^2}{n} - \left ( \dfrac{\sum x_2}{n} \right)^2 }$

$= \sqrt{\dfrac{7769}{20} - \left ( \dfrac{383}{20} \right)^2}$

$= \sqrt{21.7275}$

$= 4.66 \text{cm}$

---

About the Textbook:

Name: Basic Mathematics Grade XI

Author(s): D.R. Bajracharya | R.M. Shresththa | M.B. Singh | Y.R. Sthapit | B.C. Bajracharya

Publisher: Sukunda Pustak Bhawan (Bhotahity, Kathmandu)

Telephone: 5320379, 5353537

Price: 695 /- (2078 BS)

Website: Sukunda Publication – Sukunda Pustak Bhawan

Buy this book: Basic Mathematics : Grade XI – Sukunda Publication